Free PDF Download of CBSE Class 10 Maths Chapter 9 Application of Trigonometry Multiple Choice Questions with Answers. MCQ Questions for Class 10 Maths with Answers was Prepared Based on Latest Exam Pattern. Students can solve NCERT Class 10 Maths Application of Trigonometry MCQs with Answers to know their preparation level.
Applications Of Trigonometry Word Problems Worksheet Answers October 3, 2020 by admin 21 Posts Related to Applications Of Trigonometry Word Problems Worksheet Answers. Applications of Trigonometry. Land Surveyor using Theodolite (MCQs) Q1: When a point is observed, the angle formed by the line of sight with the horizontal level.
Class 10 Maths MCQs Chapter 9 Application of Trigonometry
Some Applications Of Trigonometry Class 10 Answers
Trigonometric formulae are useful for solving problems in two dimensions. However, in the real world all objects are three dimensional, so it is important that we extend the application of the area, sine and cosine formulae to three dimensional situations. Drawing a three dimensional diagram is a crucial step in finding the solution to a problem. Applications Of Right Triangle Trigonometry Answers We can also use right triangles to find distances using angles given as bearings. In navigation, a bearing is the direction from one object to another. In air navigation, bearings are given as angles rotated clockwise from the north.
MCQ On Application Of Trigonometry Class 10 Question 1. The shadow of a tower is equal to its height at 10-45 a.m. The sun’s altitude is
(a) 30°
(b) 45°
(c) 60°
(d) 90°
Answer: b
Explaination: Reason: Let the height of tower BC = rm and sun’s altitude = θ
Then Length of its shadow, AB = x m
In rt. ∆ABC, tan θ = (frac{BC}{AB}) = (frac{x}{x}) = 1
⇒ tan θ = tan 450
∴ θ = 45°
2. In given figure, the value of CE is
(a) 12 cm
(b) 6 cm
(c) 9 cm
(d) 6√3 cm
Answer: a
Explaination: Reason: In rt. ∆EBC, cos 60° = (frac{BC}{CE})
⇒ (frac{1}{2}) = (frac{6}{CE})
⇒ CE = 12 cm
MCQ Questions For Class 10 Maths Trigonometry Question 3. In given figure, the value of ZC is
(a) 90°
(b) 45°
(c) 30°
(d) 60°
Answer: d
Explaination: Reason: In rt. ∆ABC, cos C = (frac{BC}{AB}) = (frac{7}{14}) = (frac{1}{2})
⇒ cos C = cos 60°
∴ C = 60°
MCQ On Application Of Trigonometry Question 4. In given Fig., the angle of depression from the observing position D and E of the object at A are
(a) 60°, 60°
(b) 30°, 30°
(c) 30°, 60°
(d) 60°, 30°
Answer: c
Explaination:
Reason: ∵ APD, ∠1 = 90° – 60° = 30°
∴ APE, ∠2 = ∠EAB …[alt Zs]
∴ ∠2 = 60°
Hence the angles of depression at D and E are 30° and 60° respectively.
Some Applications Of Trigonometry Class 10 MCQ Question 5. In given figure, the length of AP is
Answer: b
Explaination:
MCQ NCERT Application Of Trigonometry Class 10 Question 6. In given figure, the value of AE is
Answer: a
Explaination: Reason: ∠AED = ∠EAB = 30°
In rt. ∆AED, sin 30° = (frac{AD}{AP})
⇒ (frac{1}{2}) = (frac{45}{AE})
⇒ AE = 90 cm
Trigonometry MCQ Class 10 Question 7. In given figure, AD = 4 cm, BD = 3 cm and CB = 12 cm. The value of tan θ is
Answer: c
Explaination: Reason: In rt ∆ADB,
AB² = AD² + BD² = (4)² + (3)² = 16 + 9 = 25
∴ AB = √25 = 5
∴ In rt ∆ABC, tan θ (frac{AB}{BC}) = (frac{5}{12})
8. In figure given ABCD is a rectangle, the value of CE is
(a) 1 cm
(b) 2 cm
(c) 3 cm
(d) 4 cm
Answer: d
Explaination: Reason: Since ABCD is a rectangle
∴ BC = AD = 8 cm and B = 90°
In rt ∆CBE, cos 60° = (frac{CE}{BC})
⇒ (frac{1}{2}) = (frac{CE}{8})
∴ CE = (frac{8}{2}) = 4 cm
Trigonometry MCQ Questions Question 9. In given figure, ABCD is a || gm. The lenght of AP is
(a) 2 cm
(b) 4 cm
(c) 6 cm
(d) 8 cm
Answer: c
Explaination: Reason: Since ABCD is a || gm
∴ AD = BC = 4√3
In rt ∆APD, sin 60° = (frac{AP}{AD})
⇒ (frac{sqrt{3}}{2}=frac{mathrm{AP}}{4 sqrt{3}})
⇒ 2AP = 4 × 3 = 12
∴ AP = 6 cm
MCQ On Trigonometry For Class 10 With Solutions Question 10. When the length of shadow of a vertical pole is equal to √3 times of its height, the angle of elevation of the Sun’s altitude is
(a) 30°
(b) 45°
(c) 60°
(d) 15°
Answer: a
Explaination: Reason: Let the height of the vertical pole, BC = h m
∴ Shadow AB = √3 h m and the angle of elevation ZBAC = θ
In rt ∆ABC, tan θ = (frac{B C}{A B}=frac{h}{sqrt{3} h}=frac{1}{sqrt{3}}) = tan 30°
∴ θ = 30°
Hence the Sun’s altitude is 30°
MCQ Trigonometry Class 10 Question 11. The angle of elevation of top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30°. The length of the tower is
(a) √3 m
(b) 2√3 m
(c) 5√3m
(d) 10√3 m
Answer: d
Explaination:
MCQ Questions On Trigonometry For Class 10 Question 12. A plane is observed to be approaching the airport. It is at a distance of 12 km from the point of observation and makes an angle of elevation of 60°. The height above the ground of the plane is
(a) 6√3 m
(b) 4√3 m
(c) 3√3 m
(d) 2√3 m
Answer: a
Explaination:
Trigonometry Multiple Choice Questions And Answers Pdf Question 13. The upper part of a tree is broken by the wind and makes an angle of 30° with the ground. The distance from the foot of the tree to the point where the top touches the ground is 5 m. The height of the tree is
(a) 10√33 m
(b) 5√33 m
(c) √3 m
(d) √3/5 m
Answer: b
Explaination:
MCQ On Trigonometry 10th Question 14. The angles of elevation of the top of a rock from the top and foot of 100 m high tower are respectively 30° and 45°. The height of the rock is
(a) 50 m
(b) 150 m
(c) 5o√3m
(d) 50(3 + √3)
Answer: d
Trigonometry MCQs Question 15. The tops of two poles of height 20 m and 14 m are connected by a wire. If the wire makes an angle of 30° with horizontal, the length of the wire is
(a) 6 m
(b) 10 m
(c) 12 m
(d) 20 m
Answer: c
We hope the given MCQ Questions for Class 10 Maths Application of Trigonometry with Answers will help you. If you have any query regarding CBSE Class 10 Maths Chapter 9 Application of Trigonometry Multiple Choice Questions with Answers, drop a comment below and we will get back to you at the earliest.
Get Free NCERT Solutions for Class 10 Maths Chapter 9 Ex 9.1 PDF. Some Applications of Trigonometry Class 10 Maths NCERT Solutions are extremely helpful while doing your homework. Exercise 9.1 Class 10 Maths NCERT Solutions were prepared by Experienced LearnCBSE.in Teachers. Detailed answers of all the questions in Chapter 9 Maths Class 10 Some Applications of Trigonometry Exercise 9.1 provided in NCERT TextBook.
Applications Of Trigonometry Questions And Answers
Topics and Sub Topics in Class 10 Maths Chapter 9 Some Applications of Trigonometry:
| Section Name | Topic Name |
| 9 | Some Applications of Trigonometry |
| 9.1 | Introduction |
| 9.2 | Heights And Distances |
| 9.3 | Summary |
NCERT Solutions For Class 10 Maths Chapter 9 Some Applications of Trigonometry Ex 9.1
NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry Ex Ex 9.1 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry Exercise 9.1.
| Board | CBSE |
| Textbook | NCERT |
| Class | Class 10 |
| Subject | Maths |
| Chapter | Chapter 9 |
| Chapter Name | Some Applications of Trigonometry |
| Exercise | Ex 9.1 |
| Number of Questions Solved | 16 |
| Category | NCERT Solutions |
Hi all, We have also solved 68 questions of Chapter 12 – Some Applications of Trigonometry from RD Sharma Class 10 Maths textbook. You can download these solutions in PDF from the above link.
Ex 9.1 Class 10 Maths Question 1.
A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30°.
Solution:
Ex 9.1 Class 10 Maths Question 2.
A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree.
Solution:
You can also download the free PDF of Ex 9.1 Class 10 Some Applications of Trigonometry NCERT Solutions or save the solution images and take the print out to keep it handy for your exam preparation.
Ex 9.1 Class 10 Maths Question 3.
A contractor plans to install two slides for the children to play in a park. For the children below the age of 5 years, she prefers to have a slide whose top is at a height of 1.5 m, and is inclined at an angle of 30° to the ground, whereas for elder children, she wants to have a steep slide at a height of 3 m, and inclined at an angle of 60° to the ground. What should be the length of the slide in each case?
Solution:
Ex 9.1 Class 10 Maths Question 4.
The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30°. Find the height of the tower.
Solution:
Applications Of Trigonometry Class 10 Answers
Ex 9.1 Class 10 Maths Question 5.
A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60°. Find the length of the string, assuming that there is no slack in the string.
Solution:
Ex 9.1 Class 10 Maths Question 6.
A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increases from 30° to 60° as he walks towards the building. Find the distance he walked towards the building.
Solution:
Ex 9.1 Class 10 Maths Question 7.
From a point on the ground, the angles of elevation of the bottom and the top of a transmission tower fixed at the top of a 20 m high building are 45° and 60° respectively. Find the height of the tower.
Solution:
Ex 9.1 Class 10 Maths Question 8.
A statue, 1.6 m tall, stands on the top of a pedestal. From a point on the ground, the angle of elevation of the top of the statue is 60° and from the same point the angle of elevation of the top of the pedestal is 45°. Find the height of the pedestal.
Solution:
Ex 9.1 Class 10 Maths Question 9.
The angle of elevation of the top of a building from the foot of a tower is 30° and the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.
Solution:
Ex 9.1 Class 10 Maths Question 10.
Two poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60° and 30° respectively. Find the height of the poles and the distance of the point from the poles.
Solution:
Ex 9.1 Class 10 Maths Question 11.
A TV tower stands vertically on a bank of a canal. From a point on the other bank directly opposite the tower, the angle of elevation of the top of the tower is 60°. From another point 20 m away from this point on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is 30° (see the given figure). Find the height of the tower and the width of the CD and 20 m from pole AB.
Solution:
Ex 9.1 Class 10 Maths Question 12.
From the top of a 7 m high building, the angle of elevation of the top of a cable tower is 60° and the angle of depression of its foot is 45°. Determine the height of the tower.
Solution:
Ex 9.1 Class 10 Maths Question 13.
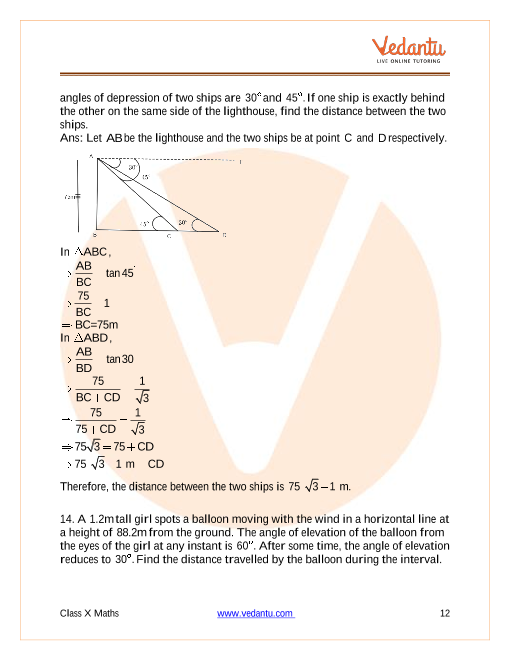
As observed from the top of a 75 m high lighthouse from the sea-level, the angles of depression of two ships are 30° and 45°. If one ship is exactly behind the other on the same side of the lighthouse, find the distance between the two ships.
Solution:
Ex 9.1 Class 10 Maths Question 14.
A 1.2 m tall girl spots a balloon moving with the wind in a horizontal line at a height of 88.2 m from the ground. The angle of elevation of the balloon from the eyes of the girl at any instant is 60°. After sometime, the angle of elevation reduces to 30° (see figure). Find the distance travelled by the balloon during the interval.
Solution:
Ex 9.1 Class 10 Maths Question 15.
A straight highway leads to the foot of a tower. A man standing at the top of the tower observes a car at an angle of depression of 30°, which is approaching the foot of the tower with a uniform speed. Six seconds later, the angle of depression of the car is found to be 60°. Find the time taken by the car to reach the foot of the tower from this point.
Solution:
Ex 9.1 Class 10 Maths Question 16.
The angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6 m.
Solution:
Applications Of Right Triangle Trigonometry Answers
Class 10 Maths Some Application Of Trigonometry Mind Maps

SOME APPLICATION OF TRIGONOMETRY
Introduction
The height or length of an object or the distance between two distinct objects can be determined with the help of trigonometric ratios.
Line of Sight and Angle of Elevation
In the above figure, the line AC drawn from the eye of an observer at A to the top of the pole ‘C’ is called the line of sight. The observer is looking at the top of the pole. The angle BAC, so formed by the line of sight with the horizontal, is called the angle of elevation of the top of the pole from the eye of an observer.
Angle of Depression
In the above figure, the line AC, is the line of sight as the observer is looking downwards from the top of the building at A towards the object at C. Here angle DAC, so formed by the line of sight with the horizontal, when the observer is lowering his/her head is called Angle of depression.
From the above figure, if we want to find the height CD of the pole without actually measuring it, we need the following information:
(i) Distance ED of the observer from the pole.
(ii) the angle of elevation ∠BAC, of the top of the pole.
(iii) the height AE of the observer if it is considerable.
Assuming that the above three conditions are known we can determine the height of the pole in the following way.
In the figure, CD = CB + BD. Here, BD = AE, which is the height of the observer.
To find BC, we will use trigonometric ratios of ∠BAC or ∠A.
In ∆ABC, the side BC is the opposite side to the known ∠A. Now we use either tan A or cot A, as these trigonometric ratios involve AB and BC to find BC.
Therefore, tan A = (frac{B C}{A B}) or cot A = (frac{A B}{B C}), which on solving would give us BC. By adding AE to BC, you will get the height of the pole.
NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry (Hindi Medium) Ex 9.1
NCERT Solutions for Class 10 Maths
We hope the NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry Ex 9.1, help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry Exercise 9.1, drop a comment below and we will get back to you at the earliest.